1 How much force is used to accelerate the train across the first 42.6 m?
2 How many g's does the rider feel as he is pushed by the initial accelerating force?
3 How fast is the car traveling at location A if it coasted down the back side of the first hill?
4 At location B the incline is 90° of a circle. How many g's does the rider feel as he enters the loop at location "B?"
5 How fast is the car traveling when it reaches location "C"?
6 In the banked turn the rider is traveling 21.6 m/s. What is the optimum angle of the banked curve?
7 How many g's does the rider feel as he enters the loop?
8 What is the height of the loop?
9 If the radius at the top of the loop is 7.45 m, then how many g's does the rider feel at this location?
10 How high does the coaster train coast at the end of the track?

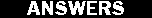
1 Use energy relationships to solve
ET_(BEGINNING) + WORK = ET_(BEGINNING)
0 + Fd = (¹/2) mv²
F(42.6) = (¹/2) 5634)(38.6)²
F(42.6) = 4197217.32
F = 98,526.22817 N
F = 98,500 N

2 Use kinematics to solve
v_f² = v_o² + 2ax
38.6² = 0 + 2a(42.6)
a_c = 17.48779 ^m/s²


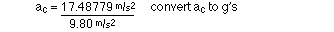
3 Use energy relationships to solve
ET_{(AFTER FORCE)} = ET _{(at A)}
KE + PE = KE + PE
(¹/2)mv² + mgh = (¹/2)mv² + 0
(¹/2)v² + gh = (¹/2) v² + 0
(¹/2)(36.8)² + 9.8(12.1) = (¹/2)v²
863.56 = (¹/2)v²
1727.12 = v²
v = 41.55863 ^m/s
v = 41.6 ^m/s

4 v = 45.5 m/s, r = 70.3 m


g's felt at the bottom = a_c[in g's] + 1 g
g's felt at the bottom = 3.0049787 + 1 g
g's felt at the bottom = 4.00 g's


5 Use energy relationships and compare locations "B" with "C"
ET_B = ET_C
KE + PE = KE + PE
(¹/2)mv² + 0 = (¹/2)mv² + mgh
(¹/2)v² + 0 = (¹/2)v² + gh
(¹/2)(45.5)² + 0 = (¹/2)v² + 9.8(61.3)
1035.125 = (¹/2)v² +600.74
868.77 = v²
v = 29.4749 ^m/s
v = 29.5 ^m/s

6 Use banked curve equations
v = 21.6 ^m/s, r = 42.3 m

7 Use circular motion equations
v = 45.5 ^m/s, r = 35.3 m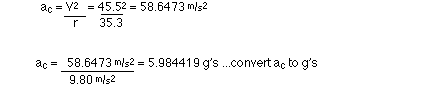


g's felt at the bottom = a_c[in g's] + 1 g
g's felt at the bottom = 5.984419 + 1 g
g's felt at the bottom = 6.98 g's

8 Use energy relationships
v_(BOTTOM) = 45.5 ^m/s, V_(TOP) = 15.2 ^m/s
ET_(TOP) = ET_(BOTTOM)
KE + PE = KE + PE
(¹/2)mv² + mgh = (¹/2)mv² + 0
(¹/2)v² + gh = (¹/2)v²
(¹/2)(15.2)² + (9.80)h = (¹/2)(45.5)²
115.52 + 9.8(h) = 1035.125
h =93.8372 m
h = 93.8 m


9 Use circular motion relationships
v = 15.2 ^m/s, r = 7.45 m

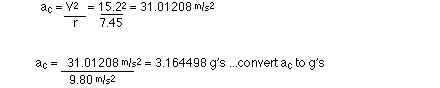
g's felt at the top of a loop = a_c[in g's] + 1 g
g's felt at the top of a loop = 3.164498 + 1 g
g's felt at the top of a loop = 2.16 g's

10 Use energy relationships to solve.
Velocity at the highest point will be zero, (apogee).
ET_(BOTTOM) = ET_(TOP)
KE + PE = KE + PE
(¹/2)mv² + 0 = 0 + mph
(¹/2)v² = gh
(¹/2)(45.5)² + = (9.80)h
h =105.625 m
h = 106 m