Neatness counts! If your work can not be clearly read and understood then it is of no value. Be meticulous.
Show your work and make comments as to what the distance and time physically measures.

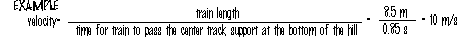
• You may get help from your notes, your text book, handouts or any other group members.
• YOU MAY NOT GET HELP FROM ANOTHER TEACHER.
• This is due the Wednesday after the trip.

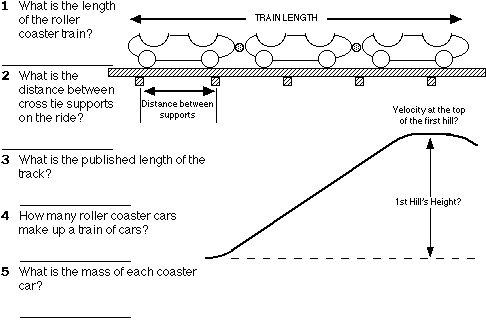
6 What is the vertical height of the 1st hill that the roller coaster car is lifted up?


7 How fast is the car traveling over the top of the hill?


8 How much time does it take for the middle of the train to reach the highest point of the first hill?


9 What is the velocity of the train at the bottom of the dip after the first hill?


10 Using the velocity in the previous question calculate the height of this drop.


If the coaster has a loop, answer questions 11-16.
11 What is the velocity of the roller coaster at the bottom of the 1st loop?


12 What is the velocity of the roller coaster at the top of the loop?


13 Using the information from the previous questions, calculate the vertical height of the loop.


14 Suppose your loop was designed using simple geometry. Below is an irregular loop shape that is designed from the splicing together of two large circles with a smaller circle. The smaller circle's radius is half the larger circle's radius. Using the information from the previous problem calculate the radii of the two circles.

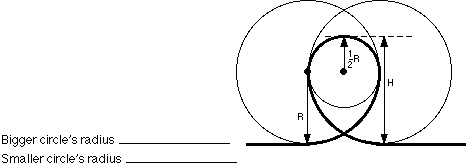
15 Using the calculated radius information above, calculate the g's felt by a rider at the bottom of the loop and at the top of the loop.




16 Using your previous answers do you think it is possible that the loop is designed according to our simple geometry model?


17 Calculate the velocity of the car as it travels over the 2nd hill.



18 Calculate the velocity of the car as it travels past the bottom of the next dip.



19 Calculate the velocity of the car as it travels over the 3rd hill.




20 Calculate the velocity of the car as it travels past the bottom of the next dip.



21 Assuming the initial total mechanical energy of the train is ZERO, how much total mechanical energy is gained by raising the train of cars to the top of the first hill.




22 The train has to lose its total mechanical energy by the time it reaches the end of the ride. Assuming 2/3's of this initial energy at the top of the hill is lost due to friction during the length of the entire ride, what is the average force of friction opposing the train's motion as it travels along the entire length of the track? (HINT: Use energy and work)







23 How much horsepower was used to raise the train up the first hill?




24 If electricity costs $0.20/(kWhr), then how much does it cost to raise the train up the first hill?




25 How many runs does a train make in 1 hour?




26 How much does it cost to run the train in a 14 hour day?